Trigonometry Story(Correct Version)
Storyboard Text
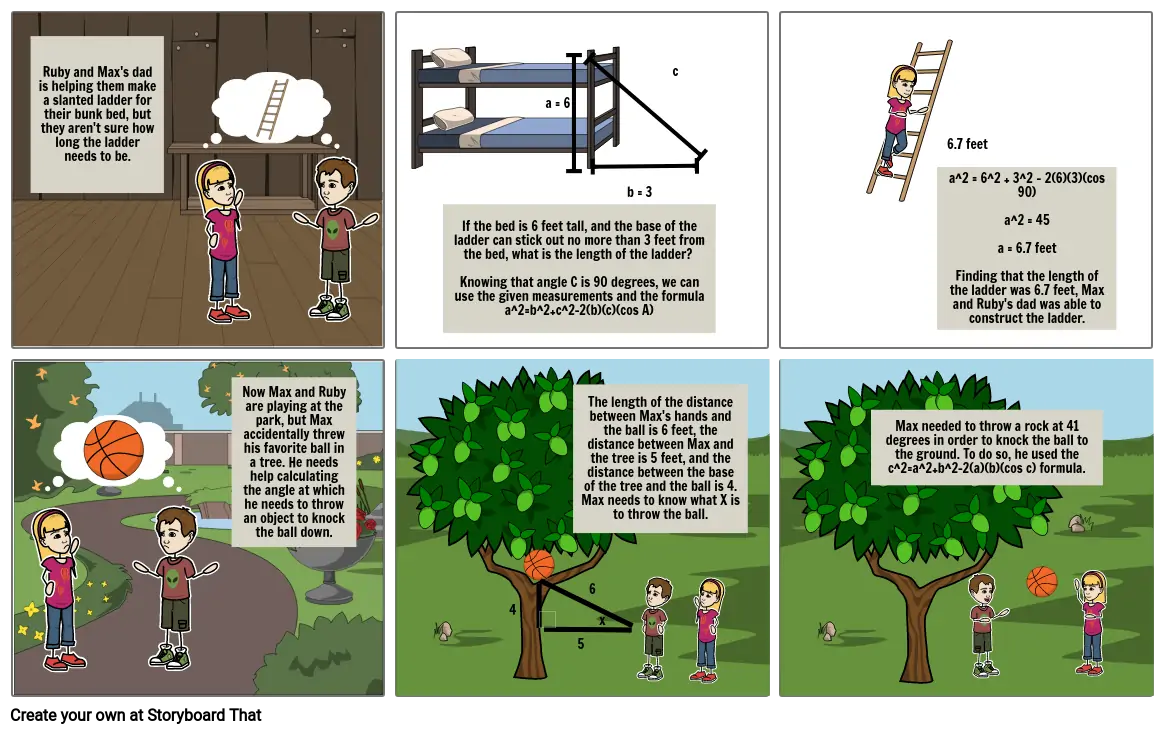
- Ruby and Max's dad is helping them make a slanted ladder for their bunk bed, but they aren't sure how long the ladder needs to be.
- .
- If the bed is 6 feet tall, and the base of the ladder can stick out no more than 3 feet from the bed, what is the length of the ladder? Knowing that angle C is 90 degrees, we can use the given measurements and the formula a^2=b^2+c^2-2(b)(c)(cos A)
- a = 6
- b = 3
- c
- 6.7 feet
- a^2 = 6^2 + 3^2 - 2(6)(3)(cos 90)a^2 = 45a = 6.7 feetFinding that the length of the ladder was 6.7 feet, Max and Ruby's dad was able to construct the ladder.
- .
- Now Max and Ruby are playing at the park, but Max accidentally threw his favorite ball in a tree. He needs help calculating the angle at which he needs to throw an object to knock the ball down.
- 4
- 5
- The length of the distance between Max's hands and the ball is 6 feet, the distance between Max and the tree is 5 feet, and the distance between the base of the tree and the ball is 4. Max needs to know what X is to throw the ball.
- 6
- x
- Max needed to throw a rock at 41 degrees in order to knock the ball to the ground. To do so, he used the c^2=a^2+b^2-2(a)(b)(cos c) formula.
Over 30 Million Storyboards Created

