Trigonometry
Text z Príbehu
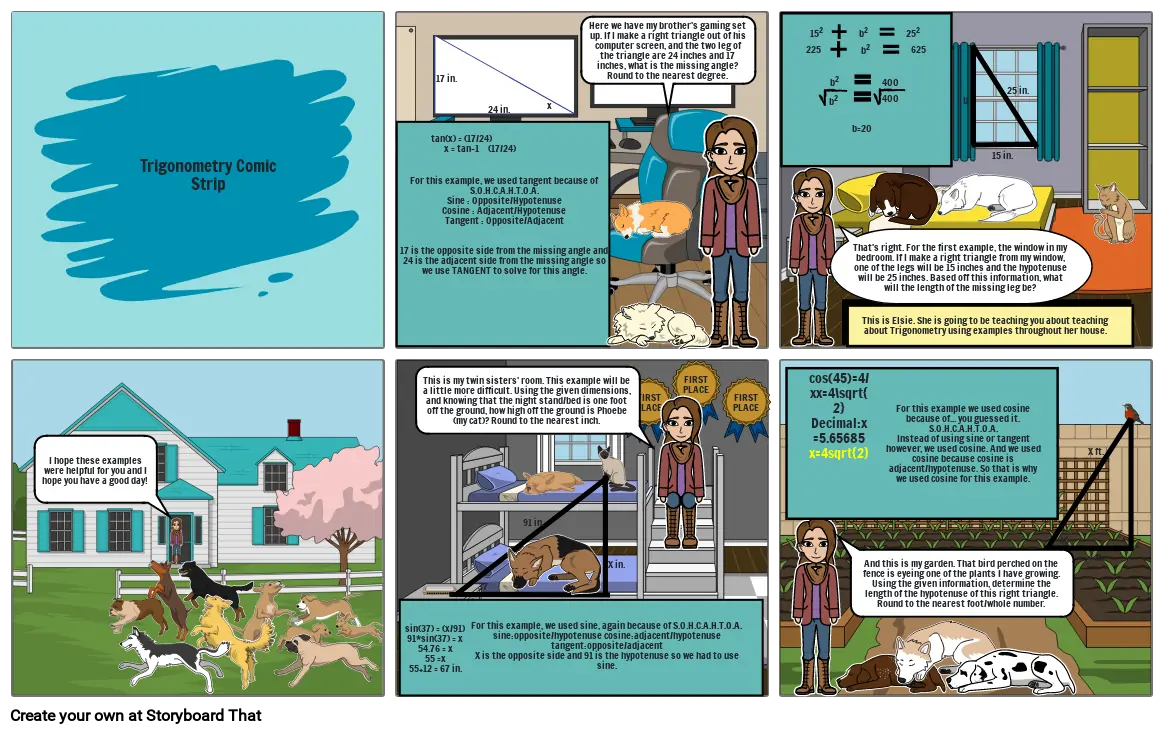
- Trigonometry Comic Strip
- For this example, we used tangent because of S.O.H.C.A.H.T.O.A. Sine : Opposite/HypotenuseCosine : Adjacent/HypotenuseTangent : Opposite/Adjacent17 is the opposite side from the missing angle and 24 is the adjacent side from the missing angle so we use TANGENT to solve for this angle.
- tan(x) = (17/24)x = tan-1
- 17 in.
- (17/24)
- 24 in.
- x
- Here we have my brother's gaming set up. If I make a right triangle out of his computer screen, and the two leg of the triangle are 24 inches and 17 inches, what is the missing angle? Round to the nearest degree.
- 225 b2 625
- 152 b2 252
- b=20
- b2
- b2
- That's right. For the first example, the window in my bedroom. If I make a right triangle from my window, one of the legs will be 15 inches and the hypotenuse will be 25 inches. Based off this information, what will the length of the missing leg be?
- This is Elsie. She is going to be teaching you about teaching about Trigonometry using examples throughout her house.
- 400
- 400
- b
- 15 in.
- 25 in.
- I hope these examples were helpful for you and I hope you have a good day!
- sin(37) = (x/91)91*sin(37) = x54.76 = x55 =x55+12 = 67 in.
- This is my twin sisters' room. This example will be a little more difficult. Using the given dimensions, and knowing that the night stand/bed is one foot off the ground, how high off the ground is Phoebe (my cat)? Round to the nearest inch.
-
- 37
-
- For this example, we used sine, again because of S.O.H.C.A.H.T.O.A.sine:opposite/hypotenuse cosine:adjacent/hypotenuse tangent:opposite/adjacentX is the opposite side and 91 is the hypotenuse so we had to use sine.
- 91 in.
- X in.
- FIRST PLACE
- FIRST PLACE
- FIRST PLACE
- cos(45)=4/xx=4\sqrt{2} Decimal:x=5.65685x=4sqrt{2}
- And this is my garden. That bird perched on the fence is eyeing one of the plants I have growing. Using the given information, determine the length of the hypotenuse of this right triangle. Round to the nearest foot/whole number.
- For this example we used cosine because of... you guessed it. S.O.H.C.A.H.T.O.A.Instead of using sine or tangent however, we used cosine. And we used cosine because cosine is adjacent/hypotenuse. So that is why we used cosine for this example.
- 45
- X ft.
- 4 ft.
Bolo vytvorených viac ako 30 miliónov storyboardov

