Unknown Story
Storyboard Tekst
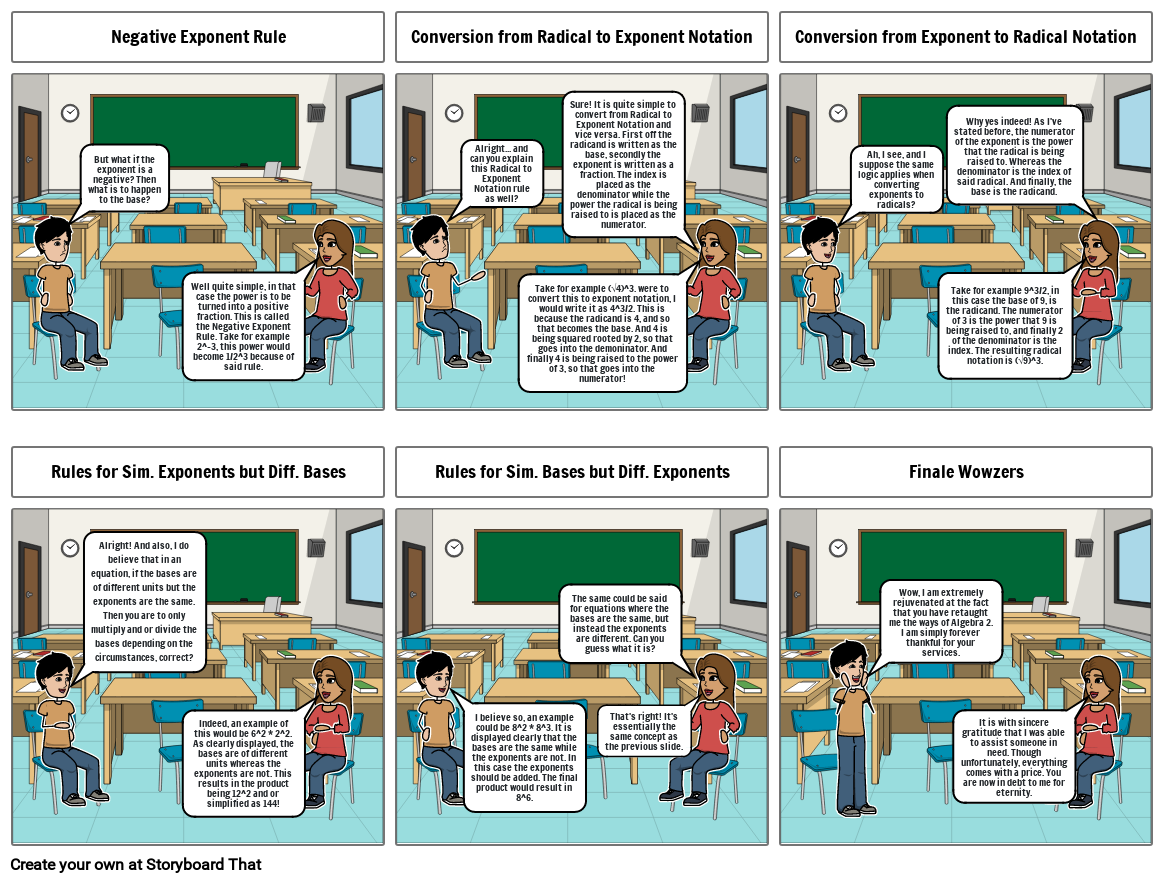
- Negative Exponent Rule
- But what if the exponent is a negative? Then what is to happen to the base?
- Well quite simple, in that case the power is to be turned into a positive fraction. This is called the Negative Exponent Rule. Take for example 2^-3, this power would become 1/2^3 because of said rule.
- Conversion from Radical to Exponent Notation
- Alright... and can you explain this Radical to Exponent Notation rule as well?
- Take for example (√4)^3. were to convert this to exponent notation, I would write it as 4^3/2. This is because the radicand is 4, and so that becomes the base. And 4 is being squared rooted by 2, so that goes into the demoninator. And finally 4 is being raised to the power of 3, so that goes into the numerator!
- Sure! It is quite simple to convert from Radical to Exponent Notation and vice versa. First off the radicand is written as the base, secondly the exponent is written as a fraction. The index is placed as the denominator while the power the radical is being raised to is placed as the numerator.
- Conversion from Exponent to Radical Notation
- Ah, I see, and I suppose the same logic applies when converting exponents to radicals?
- Take for example 9^3/2, in this case the base of 9, is the radicand. The numerator of 3 is the power that 9 is being raised to, and finally 2 of the denominator is the index. The resulting radical notation is (√9)^3.
- Why yes indeed! As I've stated before, the numerator of the exponent is the power that the radical is being raised to. Whereas the denominator is the index of said radical. And finally, the base is the radicand.
- Rules for Sim. Exponents but Diff. Bases
- Alright! And also, I do believe that in an equation, if the bases are of different units but the exponents are the same. Then you are to only multiply and or divide the bases depending on the circumstances, correct?
- Indeed, an example of this would be 6^2 * 2^2. As clearly displayed, the bases are of different units whereas the exponents are not. This results in the product being 12^2 and or simplified as 144!
- Rules for Sim. Bases but Diff. Exponents
- I believe so, an example could be 8^2 * 8^3. It is displayed clearly that the bases are the same while the exponents are not. In this case the exponents should be added. The final product would result in 8^6.
- The same could be said for equations where the bases are the same, but instead the exponents are different. Can you guess what it is?
- That's right! It's essentially the same concept as the previous slide.
- Finale Wowzers
- Wow, I am extremely rejuvenated at the fact that you have retaught me the ways of Algebra 2. I am simply forever thankful for your services.
- It is with sincere gratitude that I was able to assist someone in need. Though unfortunately, everything comes with a price. You are now in debt to me for eternity.
Over 30 millioner storyboards opprettet

