Pythagorean Theorem Comic Strip
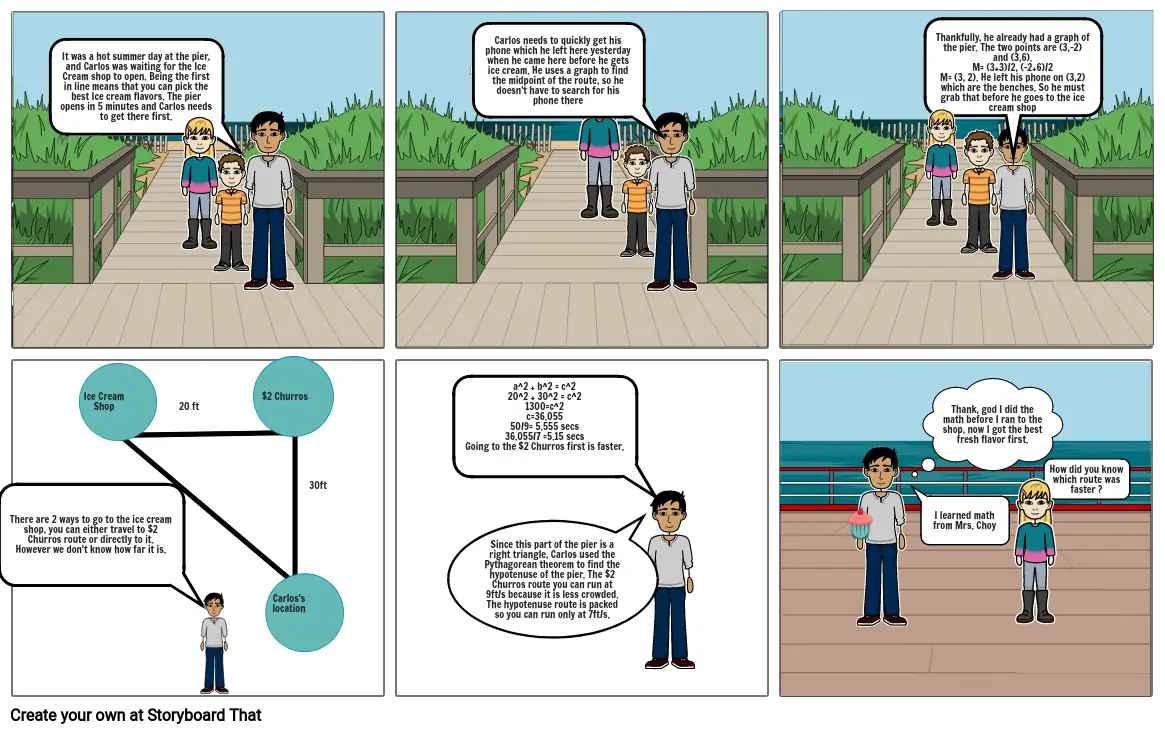
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy
It was a hot summer day at the pier, and Carlos was waiting for the Ice Cream shop to open. Being the first in line means that you can pick the best Ice cream flavors. The pier opens in 5 minutes and Carlos needs to get there first.
Thankfully, he already had a graph of the pier. The two points are (3,-2) and (3,6).
M= (3+3)/2, (-2+6)/2
M= (3, 2). He left his phone on (3,2) which are the benches. So he must grab that before he goes to the ice cream shop
$2 Churros
Carlos's
location
Ice Cream
Shop
30ft
20 ft
x
There are 2 ways to go to the ice cream shop, you can either travel to $2 Churros route or directly to it. However we don't know how far it is.
a^2 + b^2 = c^2
20^2 + 30^2 = c^2
1300=c^2
c=36.055
50/9= 5.555 secs
36.055/7 =5.15 secs
Going to the $2 Churros first is faster.
Thank, god I did the math before I ran to the shop, now I got the best fresh flavor first.
Since this part of the pier is a right triangle, Carlos used the Pythagorean theorem to find the hypotenuse of the pier. The $2 Churros route you can run at 9ft/s because it is less crowded. The hypotenuse route is packed so you can run only at 7ft/s.
Carlos needs to quickly get his phone which he left here yesterday when he came here before he gets ice cream. He uses a graph to find the midpoint of the route, so he doesn't have to search for his phone there
How did you know which route was faster ?
I learned math from Mrs. Choy