Tickets For Prom
תיאור Storyboard
This is a story of Emma and her time selling Prom tickets
טקסט Storyboard
- Tickets For Prom
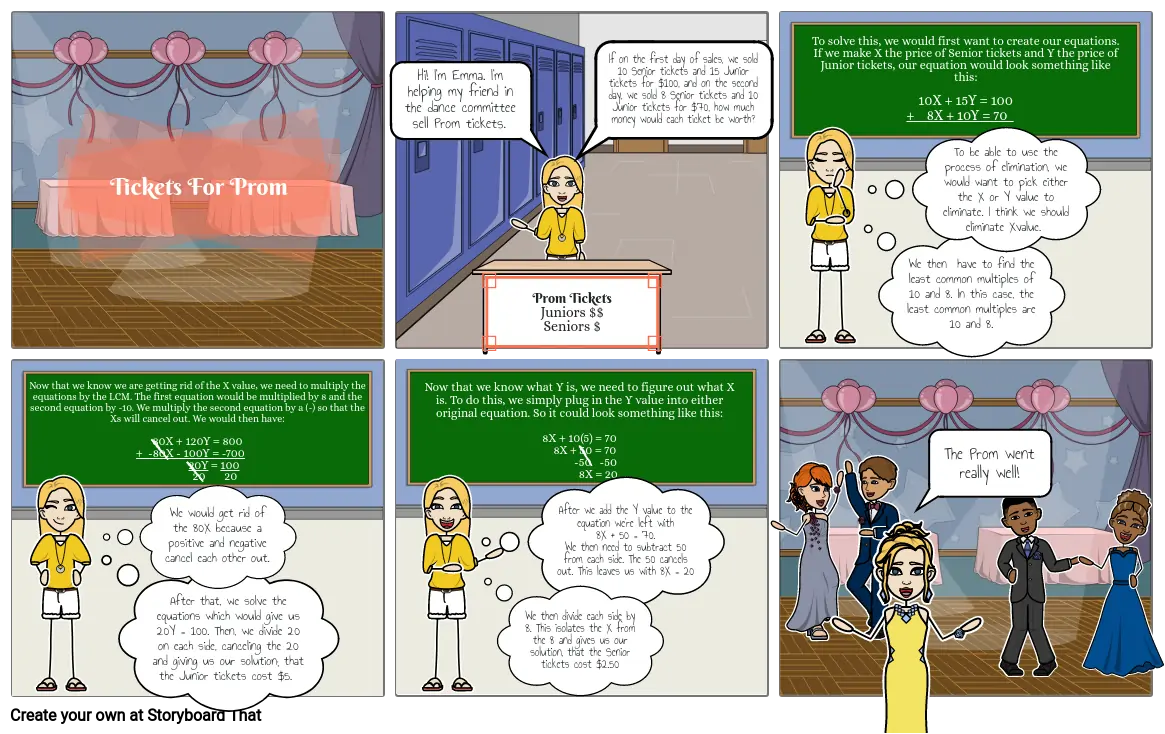
- Hi! I'm Emma. I'm helping my friend in the dance committee sell Prom tickets.
- Prom TicketsJuniors $$Seniors $
- If on the first day of sales, we sold 10 Senior tickets and 15 Junior tickets for $100, and on the second day, we sold 8 Senior tickets and 10 Junior tickets for $70, how much money would each ticket be worth?
- To solve this, we would first want to create our equations. If we make X the price of Senior tickets and Y the price of Junior tickets, our equation would look something like this:10X + 15Y = 100+ 8X + 10Y = 70
- We then have to find the least common multiples of 10 and 8. In this case, the least common multiples are 10 and 8.
- To be able to use the process of elimination, we would want to pick either the X or Y value to eliminate. I think we should eliminate Xvalue.
- Now that we know we are getting rid of the X value, we need to multiply the equations by the LCM. The first equation would be multiplied by 8 and the second equation by -10. We multiply the second equation by a (-) so that the Xs will cancel out. We would then have:80X + 120Y = 800+ -80X - 100Y = -700 20Y = 100 20 20
- After that, we solve the equations which would give us 20Y = 100. Then, we divide 20 on each side, canceling the 20 and giving us our solution; that the Junior tickets cost $5.
- We would get rid of the 80X because a positive and negative cancel each other out.
- Now that we know what Y is, we need to figure out what X is. To do this, we simply plug in the Y value into either original equation. So it could look something like this:8X + 10(5) = 70 8X + 50 = 70 -50 -50 8X = 20
- After we add the Y value to the equation we're left with 8X + 50 = 70.We then need to subtract 50 from each side. The 50 cancels out. This leaves us with 8X = 20
- We then divide each side by 8. This isolates the X from the 8 and gives us our solution, that the Senior tickets cost $2.50
- The Prom went really well!
נוצרו מעל 30 מיליון לוחות סיפור

