Trig on the Moon
Texte du Storyboard
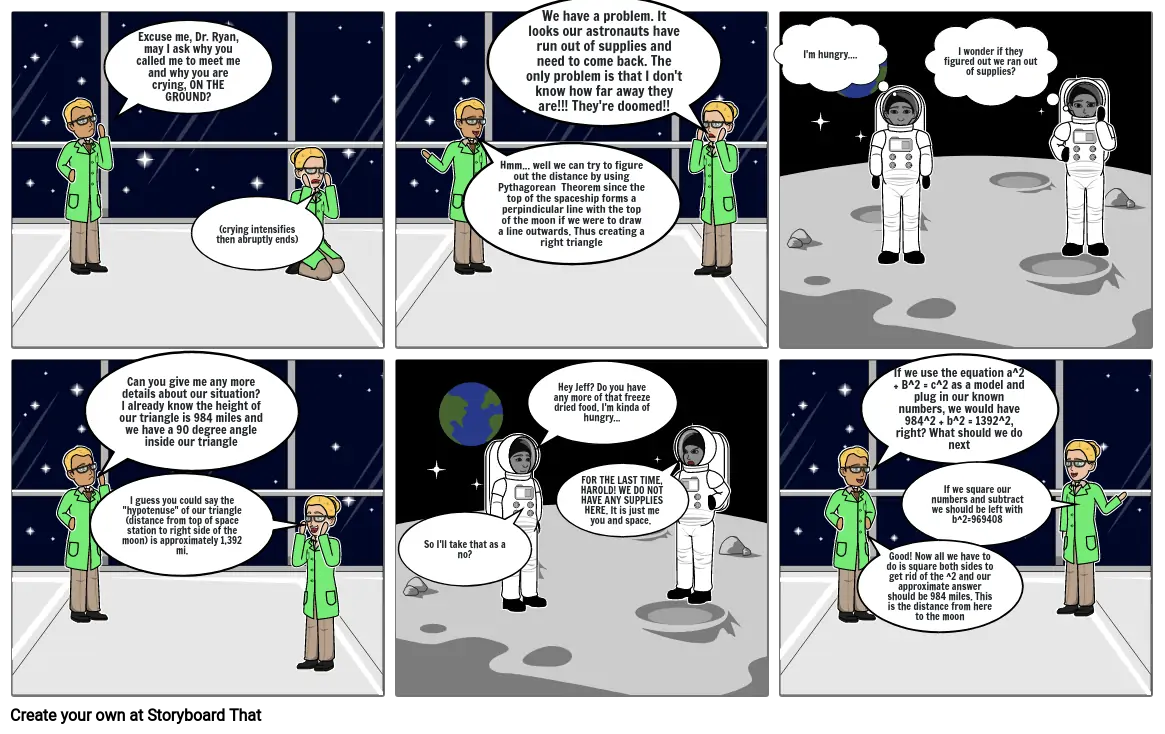
- Excuse me, Dr. Ryan, may I ask why you called me to meet me and why you are crying, ON THE GROUND?
- (crying intensifies then abruptly ends)
- Hmm... well we can try to figure out the distance by using Pythagorean Theorem since the top of the spaceship forms a perpindicular line with the top of the moon if we were to draw a line outwards. Thus creating a right triangle
- We have a problem. It looks our astronauts have run out of supplies and need to come back. The only problem is that I don't know how far away they are!!! They're doomed!!
- I'm hungry....
- I wonder if they figured out we ran out of supplies?
- Can you give me any more details about our situation? I already know the height of our triangle is 984 miles and we have a 90 degree angle inside our triangle
- I guess you could say the "hypotenuse" of our triangle (distance from top of space station to right side of the moon) is approximately 1,392 mi.
- So I'll take that as a no?
- Hey Jeff? Do you have any more of that freeze dried food. I'm kinda of hungry...
- FOR THE LAST TIME, HAROLD! WE DO NOT HAVE ANY SUPPLIES HERE. It is just me you and space.
- Good! Now all we have to do is square both sides to get rid of the ^2 and our approximate answer should be 984 miles. This is the distance from here to the moon
- If we use the equation a^2 + B^2 = c^2 as a model and plug in our known numbers, we would have 984^2 + b^2 = 1392^2, right? What should we do next
- If we square our numbers and subtract we should be left with b^2=969408
Plus de 30 millions de storyboards créés

