Trigonometry Story
Storyboard-Text
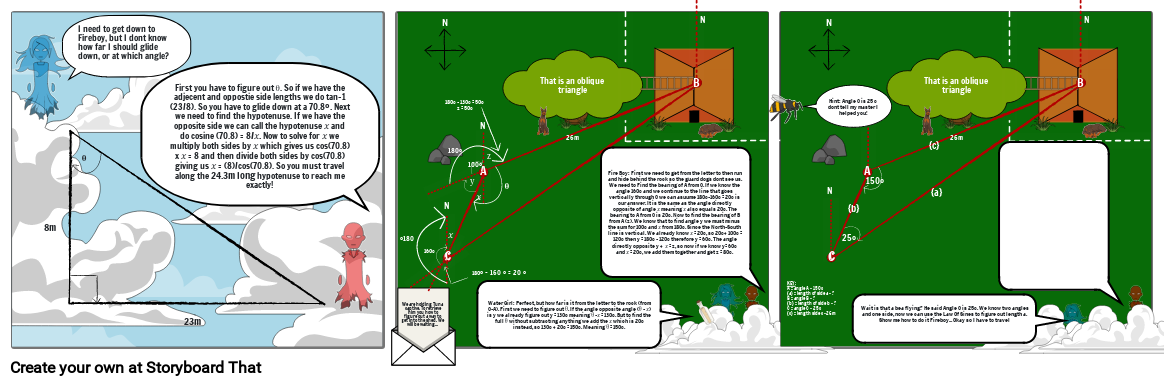
- 8m
- θ
- I need to get down to Fireboy, but I dont know how far I should glide down, or at which angle?
- First you have to figure out θ. So if we have the adjecent and oppostie side lengths we do tan-1 (23/8). So you have to glide down at a 70.8°. Next we need to find the hypotenuse. If we have the opposite side we can call the hypotenuse 𝑥 and do cosine (70.8) = 8/𝑥. Now to solve for 𝑥 we multiply both sides by 𝑥 which gives us cos(70.8) x 𝑥 = 8 and then divide both sides by cos(70.8) giving us 𝑥 = (8)/cos(70.8). So you must travel along the 24.3m long hypotenuse to reach me exactly!
- 23m
- We are holding Tuna captive. To retrieve him you how to figure out a way to get into the shed. We will be waiting...
- °180
- 160°
- N
- 𝑥
- 180° - 130° = 50°z = 50°
- C
- N
- C
- 180°
- 100°
- y
- 180° - 160 ° = 20 °
- 𝑥
- A
- N
- z
- θ
- That is an oblique triangle
- 26m
- Fire Boy: First we need to get from the letter to then run and hide behind the rock so the guard dogs dont see us. We need to Find the bearing of A from C. If we know the angle 160° and we continue to the line that goes vertically through C we can asuume 180°-160° = 20° is our answer. It is the same as the angle directly opposite of angle 𝑥 meaning 𝑥 also equals 20°. The bearing to A from C is 20°. Now to find the bearing of B from A (z). We know that to find angle y we must minus the sum for 100° and 𝑥 from 180°. Since the North-South line is vertical. We already know 𝑥 = 20°, so 20°+ 100° = 120° then y = 180° - 120° therefore y = 60°. The angle directly opposite y + 𝑥 = z, so now if we know y= 60° and 𝑥 = 20°, we add them together and get z = 80°.
- N
- B
- Water Girl: Perfect, but how far is it from the letter to the rock (from C-A). First we need to figure out θ. If the angle opposite angle (θ - 𝑥) is y we already figure out y = 130° meaning θ -𝑥 = 130°. But to find the full θ without subtracting anything we add the 𝑥 which is 20° instead, so 130° + 20° = 150°. Meaning θ = 150°.
- KEY:A = angle A - 150°{a} = length of side a - ?B = angle B - ?{b} = length of side b - ?C = angle C - 25°{c} = length side c -26m
- Hint: Angle C is 25 ° dont tell my master I helped you!
- N
- N
- C
- C
- 25°
- {b}
- A
- N
- 150°
- That is an oblique triangle
- {c}
- {a}
- 26m
- N
- Wait is that a bea flying? He said Angle C is 25°. We know two angles and one side, now we can use the Law Of Sines to figure out length a. Show me how to do it Fireboy... Okay so I have to travel
- B
Über 30 Millionen erstellte Storyboards

