Role Playing
نص القصة المصورة
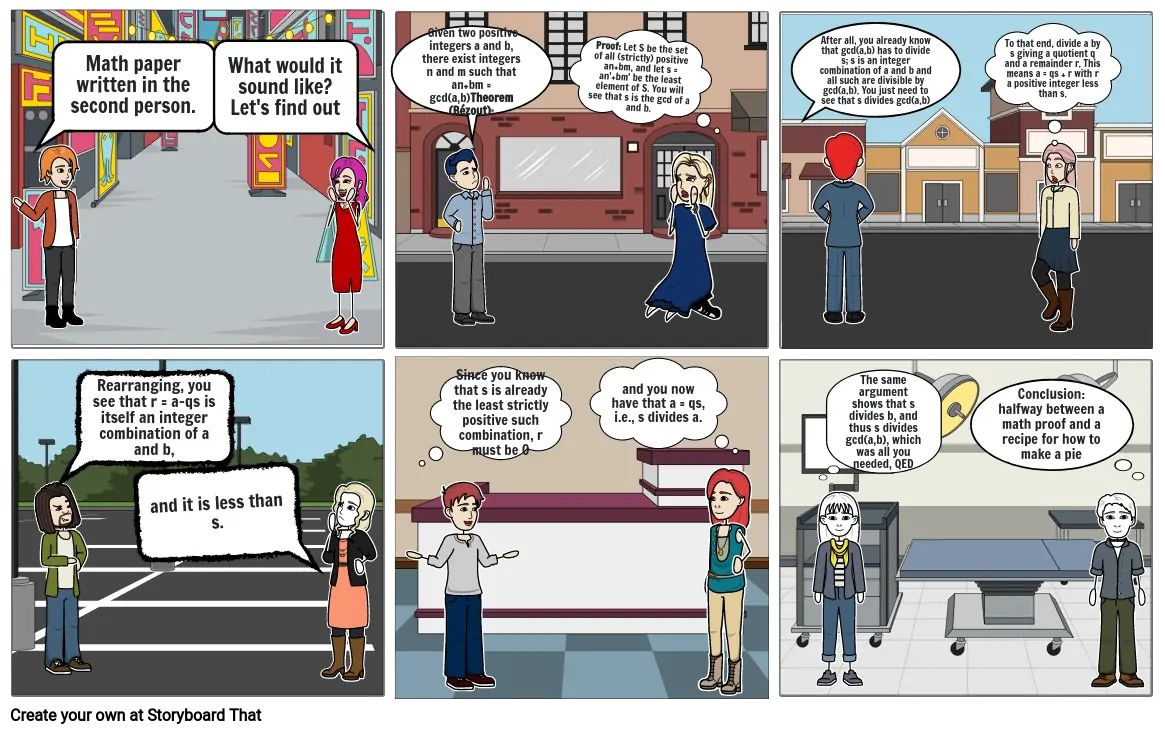
- Math paper written in the second person.
- What would it sound like? Let's find out
- Given two positive integers a and b, there exist integers n and m such that an+bm = gcd(a,b)Theorem (Bézout):
- Proof: Let S be the set of all (strictly) positive an+bm, and let s = an'+bm' be the least element of S. You will see that s is the gcd of a and b.
- After all, you already know that gcd(a,b) has to divide s; s is an integer combination of a and b and all such are divisible by gcd(a,b). You just need to see that s divides gcd(a,b)
- To that end, divide a by s giving a quotient q and a remainder r. This means a = qs + r with r a positive integer less than s.
- Rearranging, you see that r = a-qs is itself an integer combination of a and b,
- and it is less than s.
- Since you know that s is already the least strictly positive such combination, r must be 0
- and you now have that a = qs, i.e., s divides a.
- The same argument shows that s divides b, and thus s divides gcd(a,b), which was all you needed, QED
- Conclusion: halfway between a math proof and a recipe for how to make a pie
تم إنشاء أكثر من 30 مليون من القصص المصورة

